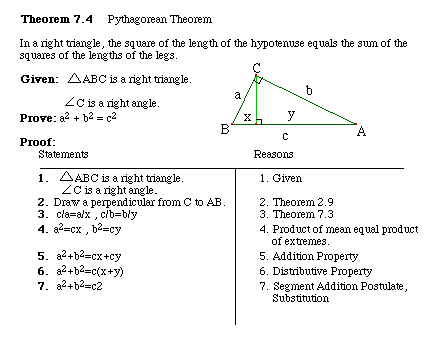
|
|
![]()
and the two-column proof format |
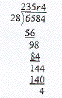
A long division. |
Just as the long division algorithm complies
with the purpose of finding a quotient and a
remainder, the two-column proof format does comply
with the task of providing a proof. But these kind
of proofs are not really offered to a community
that aims at establishing the validity of a
proposition (Balacheff, 1987, p. 147). Rather, like
long division with all its partial products and
subtractions, two-column proofs are offered to the
teacher whose agenda is neither to be convinced of
the validity of a proposition nor to be enlightened
by the ideas of the proof. The teacher's agenda is
not even to rejoice in the elegance of an argument,
but to check the degree to which the expected
sequence of steps for each proof-exercise has been
achieved. |
At a fortunate point in time in which the importance of
proof comes back to the American rhetoric on mathematics
education (see Kilpatrick, 1997; Wu, 1997; Ross, 1998; NCTM,
1998, pp. 80-85), it seems in order to discuss the nuances
around notions of proof, formal proof, two-column proof,
argumentation, and so on. My purpose is not just to indicate
that there is more to mathematical proof than the use of the
two-column proof format: That assertion may be commonplace
and the NCTM Standards (1989) had explicitly brought it to
the fore by recommending to increase attention to "deductive
arguments expressed orally and in sentence or paragraph
form" (p. 126) and to decrease attention to "two-column
proofs" (p. 127). Rather, my purpose is to outline the role
played in the practice of geometry teaching by formats such
as the two-column proof format in shaping conceptions of
geometry, reasoning, and mathematical proof. Such an outline
may afford elements for a critique of an ideologically
handy, yet objectionable, belief that relates the use of the
two-column proof format in the classroom with providing
students experiences in rigor and formalism in
mathematics.
On the one hand, the cultural imperative
that school mathematics must aim at reproducing the
conditions of production of mathematical knowledge implies
that school mathematics must afford students opportunities
to learn to prove, expose them to culturally relevant
proofs, and familiarize them with the meaning of proof in
constructing and validating mathematical knowledge. On the
other hand, students' meaningful learning of mathematics is
more likely to happen within practices that resemble those
of the production of mathematical knowledge. Insofar as
rigor and formalism serve the mathematician not only to
validate but also to construct knowledge, rigor and
formalism are natural ingredients of practices in which
mathematics can be meaningfully constructed. As the Draft
for the Standards 2000 states,
It should be stressed that exploring, conjecturing, representing, and proving are all deeply connected aspects of mathematical thinking. "Reasoning" and "Proof" should not be thought of as separable from the bulk of mathematical activity. (NCTM, 1998, p. 85)
Given the usual oversimplifications in educational
rhetoric, it seems important to note that an increased
emphasis on argumentation and mathematical proof is indeed
consistent with affording students opportunities to engage
in mathematical practice (as rigor and formalism in
mathematical practice are productive rather than
restrictive). But that increased emphasis neither entails
the need to teach two-column proofs (or any other format of
proof) explicitly nor provides a warrant that the practice
of two-column proofs will provide experiences in
mathematical rigor or formalism.
As a first step toward making a more
general argument about the particular relations between
proof, formalism, and argumentation, a longer version of
this article (Herbst,
1999) discusses some conjectures on the historical
circumstances that gave shape to the practice of two-column
proofs in the history of mathematics teaching in the United
States. The place of two-column proofs in the practice of
teaching and learning mathematics has developed in
interaction with some objects of discourse such as proof,
geometry, and reasoning. The specific characteristics of
those interactions partially account for the endurance of
the two-column-proof format and for its possible association
with rigor and formalism.
The first chapters of a history of proof
in American high school mathematics education are located in
the study of geometry. The longer version of this article
traces the changes of the notion of (what was meant by)
studying geometry as witnessing the changing conceptions of
mathematical proof, reasoning, and geometry itself. The
reasons for the endurance of the two-column-proof format are
to be found not in the circumstances of its punctual origin
(such as when it was invented and by whom) but in the
intrinsic characteristics of the practices that it came to
serve and the practices that its presence made available.
The following claims obtain support from the historical
search documented in the longer version of this paper.
Some Claims on the Two-Column Proof Format
Based on observation of current practice of proof writing
in high school, I concluded elsewhere (Herbst, 1998, pp.
239, 271) that the two-column proof format works in
solidarity with a clear division of the discursive labor of
student and teacher. The format affords teachers an implicit
criterion to indicate what can be said (hence, it leaves
unquestioned the control over epistemic operators such as
know, need, can say, etc.). The format makes students
accountable for producing an ordered list of "categorical"
statements about a given figure, whereas it reserves to the
teacher the decision as to whether the produced list of
statements constitutes a valid argument.
Those observations can explain why the
two-column proof format may be better adapted to the logic
or practice of geometry teaching than other (less regulated)
forms of argumentation. From the perspective of the teacher,
this format presents an efficient tool to ensure and control
the production of an acceptable proof by the student. From
the perspective of the student, the format does not make him
or her accountable for the construction or the explanation
of the geometric objects of knowledge or for the
manufacturing of an argument. Instead, it requires him or
her to produce empirical observations about the figure at
hand.
The two-column proof format also bounds
what can be given to prove in the sense that it shapes the
conceptions of what geometry is. Two-column proofs work best
within a conception of geometry as the study of figures
which have already been constructed and are always
constructible. The study of geometry conceived in that way
is a descriptive study governed by a general logic that
emphasizes the logical connection between factual
properties. To be clear, Euclidean geometry is indeed all of
that but of necessity not just that. The practices unfolded
around the two-column proof format seem to discourage a
complementary conception of geometry that was central in
Euclid's Elements and in the notions of Greek geometry
(Caveing, 1990): Geometry is also the study of the
conditions that make the figures constructible. In the study
of geometry conceived in the latter way, the logical links
between statements are pondered with respect to the
substantive strength of the links: What makes a proposition
valuable is not just that a proof exists but also that
without a proof one cannot really know whether the assertion
is true (because there is such a leap from the hypothesis to
the conclusion). As the practice of two-column proofs needs
a given figure and given statements of what is given and
what is to prove, the conception of geometry as the study of
necessary and sufficient conditions is deemphasized (and one
can understand why students may proceed by trial and error
in construction problems after having proved a statement
that entails the construction procedure -- see Schoenfeld,
1988).
The two-column proof format bounds the conception of
mathematical proof and of proving in mathematics. By
separating the source of the statements that are formulated
from the arguments that are made about them, this format
emphasizes the role of proof as a certification method,
separated from the search for knowledge or the construction
of the mathematical objects of knowledge. By displacing the
statement from the proof and enabling a policing of the
statements that make up a proof, the two-column proof format
breaks the dialectic between formulation and validation
described by Lakatos (1976; see also Balacheff, 1991) and
its underlying tension between interest to know and validity
of knowledge.
As a consequence of the previous
observations, it can also be argued that the practices
associated with the two-column proof format bypass the
epistemological characteristics of mathematical reasoning.
These practices foster a reduction of mathematical reasoning
to an activity involving the psychology of the reasoning
agents and the "natural" language with which they relate to
a "given" mathematical world: Mathematical reasoning becomes
just (general) logical reasoning applied to mathematical
objects, whose conditions of existence are taken as given.
Of course, mathematical reasoning certainly involves logical
reasoning, yet what is problematic is the exclusion of an
epistemological aspect specific to the mathematical objects
being reasoned with and about. By identifying the logical
precedence of "reasons" with their temporal precedence in
the text being studied, mathematical reasoning becomes more
of an activity of describing a "given" mathematical world
than one of constructing a "possible" mathematical world (or
mathematizing). Some consequences as to the value of
mathematics in general education and to the nature of the
transfer (of the study of mathematics to other situations)
can be envisioned (see Judd, 1928; Skovsmose, 1992;
Vygotsky, 1934/1986, pp. 146-209): Mathematical reasoning
becomes suitable to reason about objects that are purely
mathematical or that have already been mathematized
elsewhere (such as the objects of the hard sciences), but is
hardly deemed apt to reason about other objects (such as
those of the soft sciences or ordinary life) beyond the
level of appearances. By fostering the notion of proof as
just logical reasoning about objects that are already
mathematical, the two-column proof format collaborates to
stop the scientific dialectic between empiricism and
rationalism in the construction of mathematical objects of
discourse (see Skovsmose, 1992, p. 6).
A Provisional Conclusion
The longer version of this article (Herbst,
1999) has proposed some conjectures regarding what
historical circumstances permitted the two-column proof
format to emerge and endure during the first half of the
century in the United States. The two-column proof format
itself did not remain unchanged, but adapted to fit the
changing characteristics of the logic of practice that it
served. Assuming those historical conjectures are plausible,
I have argued that the interaction between (changing forms
of) the two-column proof format and (changing conditions on
the) study of geometry have contributed significantly to
shape a conception of (school) geometry as the descriptive
study of figures, a conception of mathematical proof as just
a method for knowledge-certification, and a conception of
mathematical reasoning as just logical reasoning about
"given" mathematical objects of knowledge.
These plausible effects, and not the
physical form of two-column proofs are what is of interest:
As one may note, current textbooks sometimes induce the
students to write proofs in other formats as well (such as
paragraph- or flow-proofs; see Rubinstein et al., 1995, p.
396) but what has been said about the two-column proof
format could be said about the explicit teaching of
alternative formats as well.
From the perspective of the reader, the
finished product looks like an argument that validates the
proposition stated. A closer look shows that its production
may not have any more meaning than the protocols used by
lawyers or notaries, as indeed the division of labor in the
practice that produces the proof does not look like the
division of labor among a group of mathematicians
manufacturing a mathematical argument. Two-column proofs can
be called formal, but their formalism has little to do with
the productive kind of rigor and formalism that helps
mathematicians advance human understanding of mathematics
(Thurston, 1995). Those formats play an important role in
the logic of practice of geometry teaching, but they do not
necessarily involve students in experiences with
mathematical rigor and formalism.
The reactions to the contribution of Patricio Herbst will
be
published in the March/April 99 Proof Newsletter
References
Balacheff, N. (1987). Processus de preuve et situations de validation [Processes of proof and situations of validation]. Educational Studies in Mathematics, 18, 147-176.
Balacheff, N. (1991). Construction et analyse d'une situation didactique: Le cas de "la somme des angles d'un triangle" [Construction and analysis of a didactic situation: The case of "the sum of the angles of a triangle"]. Journal für Matematikkdidaktik, 12, 199-264.
Caveing, M. (1990). Platon, Aristote et les hypotheses des mathematiciens [Plato, Aristotle, and the hypotheses of mathematicians]. In J.-F. Mattei (Ed.), La naissance de la raison en Grece: Actes du congres de Niceæ Mai 1987 (pp. 119-128). Paris: Presses Universitaires de France.
Herbst, P. (1998). What works as proof in the mathematics class. Unpublished doctoral dissertation, The University of Georgia, Athens.
Herbst, P. (1999). On proof, the logic of practice of geometry teaching and the two-column proof format: Some historical considerations. On-line article.
Heilbron, J. L. (1998). Geometry civilized: History, culture, and technique. Oxford: Clarendon Press.
Hilbert, D. (1971). Foundations of geometry. (L. Unger, Trans., P. Bernays, Rev.). La Salle, IL: Open Court. (Original work published in 1899)
Judd, C. H. (1928). The fallacy of treating school subjects as "tool subjects." In J. Clarke & W. Reeve (Eds.), The National Council of Teachers of Mathematics Third Yearbook: Selected topics in the teaching of mathematics (pp. 1-10). New York: Bureau of publications of Teachers College, Columbia University.
Kilpatrick, J. (1997). Confronting reform. The American Mathematical Monthly 104, 955-962
Lakatos, I. (1976). Proofs and refutations: The logic of mathematical discovery (J. Worrall & E. Zahar, Eds.). Cambridge: Cambridge University Press.
National Council of Teachers of Mathematics. (1989). Curriculum and evaluation standards for school mathematics. Reston, VA: NCTM.
National Council of Teachers of Mathematics. (1998). Principles and standards for School Mathematics: Discussion Draft Standards 2000. On-line at www.nctm.org.
Ross, K. (1998). Doing and proving: The place of algorithms and proofs in school mathematics. American Mathematical Monthly 105, 252-255.
Rubinstein, R., Craine, T., & Butts, T. (1995). Integrated mathematics 2. Evanston, IL: McDougal Littell.
Schoenfeld, A. (1988). When good teaching leads to bad results: The disasters of "well-taught" mathematics courses. Educational Psychologist 23(2), 145-166.
Sekiguchi, Y. (1991). An investigation on proofs and refutations in the mathematics classroom. Unpublished doctoral dissertation, The University of Georgia, Athens.
Skovsmose, O. (1992). Democratic competence and reflective knowing in mathematics. For the Learning of Mathematics 12(2), 2-11.
Thurston, W. (1995). On proof and progress in mathematics. For the Learning of Mathematics 15(1), 29-37.
Usiskin, Z., Hirschhorn, D., Coxford, A., Highstone, V., Lewellen, H., Oppong, N., DiBianca, R., and Maeir, M. (1997). The University of Chicago School Mathematics Project: Geometry. (2nd ed.). Glenview, IL: Scott Foresman.
Vygotsky, L. (1986). Thought and language (Alex Kozulin, Trans. & Ed.). Cambridge, MA: MIT Press. (Original work published in 1934)
Wertsch, J. V. (1991). Voices of the mind: A sociocultural approach to mediated action. Cambridge, MA: Harvard University Press.
Wu, H. S. (1997). The mathematics education reform: Why you should be concerned and what you can do. The American Mathematical Monthly 104, 946-954.
|